On the functional form of the radial acceleration relation

Download paper here

Abstract

We apply a new method for learning equations from data—Exhaustive Symbolic Regression (ESR)—to late-type galaxy dynamics as encapsulated in the radial acceleration relation (RAR). Relating the centripetal acceleration due to baryons, $g_\text{bar}$, to the total dynamical acceleration, $g_\text{obs}$, the RAR has been claimed to manifest a new law of nature due to its regularity and tightness, in agreement with Modified Newtonian Dynamics (MOND). Fits to this relation have been restricted by prior expectations to particular functional forms, while ESR affords an exhaustive and nearly prior-free search through functional parameter space to identify the equations optimally trading accuracy with simplicity. Working with the SPARC data, we find the best functions typically satisfy $g_\text{obs}\propto g_\text{bar}$ at high $g_\text{bar}$, although the coefficient of proportionality is not clearly unity and the deep-MOND limit $g_\text{obs} \propto \sqrt{g_\text{bar}}$ as $g_\text{bar} \to 0$ is little evident at all. By generating mock data according to MOND with or without the external field effect, we find that symbolic regression would not be expected to identify the generating function or reconstruct successfully the asymptotic slopes. We conclude that the limited dynamical range and significant uncertainties of the SPARC RAR preclude a definitive statement of its functional form, and hence that this data alone can neither demonstrate nor rule out law-like gravitational behaviour.

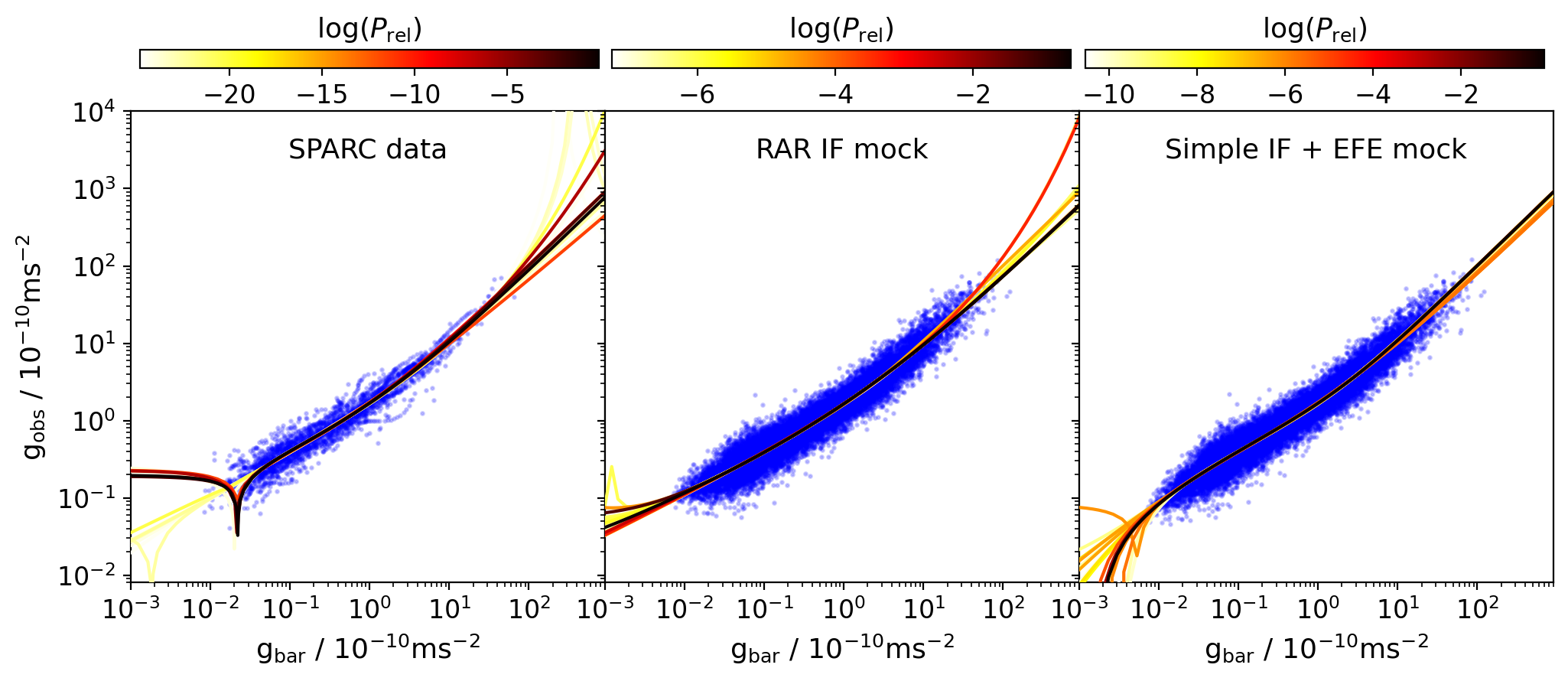The top 20 functions found by ESR overlaid on the SPARC data (blue points), colour-coded by their relative probability in the full function list. The left panel fits the SPARC data, the middle panel mock data generated by the RAR IF, and the right panel mock data generated by the Simple IF with universal external field strength $g_\text{ex}=1.2\times10^{-12}$ m s$^{-2}$. The mock datasets are $10$ times larger than SPARC, although this is factored out in the description length calculation.